Simple Explanations for the
Area and Volume Formulas

by Keith Enevoldsen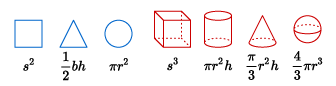

Have you memorized some of the area and volume formulas, like A = πr², without understanding the explanation for the formulas? This is an attempt to explain all the basic area and volume formulas as simply and intuitively as possible, starting with the easy ones and building up to the more difficult formulas for the area and volume of a sphere.

Areas of Plane Figures

Rectangles and Parallelograms

Area of a rectangle or parallelogram with base b and height h is bh.

	Explanation: This is obvious for a rectangle. This is the definition of the concept of area.
	A parallelogram can be cut and rearranged to form a rectangle.

Triangles

Area of a triangle with base b and height h is bh/2.

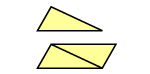Explanation: Two of the same triangles form a parallelogram.

Polygons

Area of any polygon can be determined by breaking it into simpler areas.

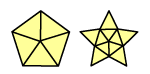Explanation: You can break any polygon into triangles.

Circles

Area of a circle is πr².

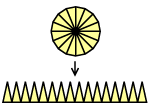Explanation: The circumference of a circle is 2πr. This is the definition of π (pi). Divide the circle into many triangular segments. The area of the triangles is 1/2 times the sum of their bases, 2πr (the circumference), times their height, r.

Surface Areas and Volumes of Solids

Prisms (including rectangular solids) and Cylinders

Lateral surface area of a right prism or cylinder with perimeter p and height h is ph. (For a cylinder, this is 2πrh.)

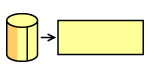Explanation: Imagine unwrapping the surface into a rectangle.

Volume of a prism or cylinder, right or oblique (slanted), with base area A and height h is Ah. (For a cylinder, this is πr²h.)

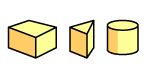	Explanation: This is obvious for a right prism or cylinder. This is the definition of the concept of volume.
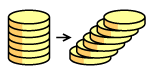	For an oblique prism or cylinder, imagine starting with a right prism or cylinder and sliding thin layers to make it oblique.

Lateral surface area of a right pyramid or cone with perimeter p and slant height s is ps/2. (For a cone, this is πrs.)

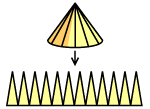Explanation: Divide the surface of the pyramid into its triangles, or the surface of the cone into many thin triangles. The area of the triangles is 1/2 times the sum of their bases, p, times their height, s.

Volume of a pyramid or cone, right or oblique (slanted), with base area A and height h is Ah/3. (For a cone, this is πr²h/3.)

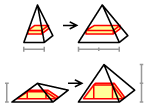	Explanation: 1. The volume is proportional to A and h. Imagine the effect of doubling A or h. Doubling A will double the volume of every horizontal slice. Doubling h will double the volume of every vertical slice.
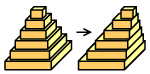	2. An oblique pyramid or cone has the same formula as a right pyramid or cone. Imagine starting with a right pyramid or cone and sliding thin layers to make it oblique.
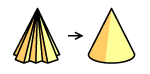	3. A cone has same formula as a pyramid. Imagine constructing an approximate cone from many thin oblique pyramids.
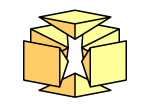	4. The constant must be 1/3. Six pyramids with 1x1 square bases and heights of 1/2 fit together (with their peaks in the center) to form a 1x1x1 cube. So the volume of one of these pyramids is 1/6, which is Ah/3.

Surface area of any polyhedron can be determined by breaking it into simpler areas.

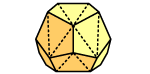Explanation: You can break the surface area of any polyhedron into triangles.

Volume of any polyhedron can be determined by breaking it into simpler solids.

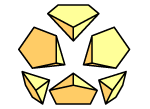Explanation: You can break a concave polyhedron into convex polyhedra, then break each convex polyhedron into pyramids with all their peaks together inside the polyhedron.

Surface area of a sphere is 4πr².

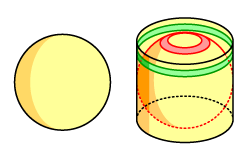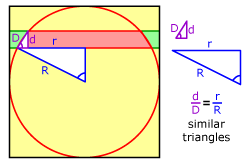Explanation: The surface area of a sphere is the same as the lateral surface area of a cylinder with the same radius and a height of 2r. The area of this cylinder is 2πrh = 2πr(2r) = 4πr2. To see why the areas are the same, first imagine the sphere sitting snugly inside the cylinder. Then imagine cutting both the sphere and cylinder at any height with two closely spaced planes which are parallel to the bases of the cylinder. Between the planes is a ring shaped strip of the cylinder's surface and a ring shaped strip of the sphere's surface. Both strips have the same area! Why? The strip of the sphere has a smaller radius but a larger width than the strip of cylinder, and these two factors exactly cancel, if the strips are very narrow. The diagram shows two right triangles. The long side of the big triangle is a radius of the sphere and the long side of the small triangle is tangent to the sphere, so the triangles meet at a right angle. You can see that the triangles are similar. This means the ratio of the radii of the two strips (r/R) is the same as the ratio of the widths of the two strips (d/D). So the areas of the strips are equal (2πRd = 2πrD). Since every strip of the sphere has the same area as the corresponding strip of the cylinder, then the area of the whole sphere is the same as the lateral area of the whole cylinder.

Volume of a sphere is 4πr³/3.

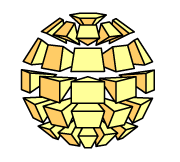Explanation: Divide the sphere into many thin pyramids with their peaks at the center. The volume of the pyramids is 1/3 times the sum of their base areas, 4πr2, times their height, r.

Historical note: Archimedes was the first to calculate the surface area and volume of a sphere.